How To Rationalize The Denominator: A Comprehensive Guide

Understanding how to rationalize the denominator is a crucial skill in algebra that can enhance your mathematical abilities. This process is essential for simplifying fractions and making them easier to work with, especially when performing operations like addition or subtraction. In this article, we will delve into the steps and techniques involved in rationalizing the denominator, ensuring that you have all the knowledge you need to tackle this topic confidently.

Rationalizing the denominator is not just a theoretical exercise; it has practical implications in various fields, including engineering, physics, and finance. By mastering this concept, you can improve your problem-solving skills and gain a deeper understanding of rational expressions. This guide will provide clear explanations, examples, and tips to help you rationalize the denominator effectively.

Throughout this article, we will cover the following topics: the definition of rationalizing the denominator, step-by-step procedures, examples of different cases, and common mistakes to avoid. By the end of this guide, you will be well-equipped to rationalize any denominator you encounter.

Table of Contents

• 1. What is Rationalizing the Denominator?
• 2. Steps to Rationalize the Denominator
• 3. Examples of Rationalizing the Denominator
• 4. Common Mistakes to Avoid
• 5. Applications of Rationalizing the Denominator
• 6. Practice Problems
• 7. Tips for Effective Rationalization
• 8. Conclusion

1. What is Rationalizing the Denominator?

Rationalizing the denominator refers to the process of eliminating any irrational numbers or radicals from the denominator of a fraction. This is often done to make calculations easier and to express the fraction in a more standard form.

For instance, a fraction like 1/√2 is not in its simplest form. By rationalizing the denominator, we can convert it into a form that avoids the square root in the denominator, which is more manageable for further calculations.

2. Steps to Rationalize the Denominator

To rationalize the denominator, follow these steps:

1. Identify the denominator: Look at the denominator of the fraction to determine if it contains an irrational number or radical.
2. Multiply by a suitable factor: Multiply both the numerator and the denominator by a factor that will eliminate the radical in the denominator.
3. Simplify the resulting fraction: After multiplication, simplify the fraction if possible.

Example 1: Rationalizing a Single Radical

Consider the fraction 3/√5. To rationalize it:

1. Multiply the numerator and denominator by √5: (3√5)/(√5√5) = 3√5/5.
2. The rationalized form is 3√5/5.

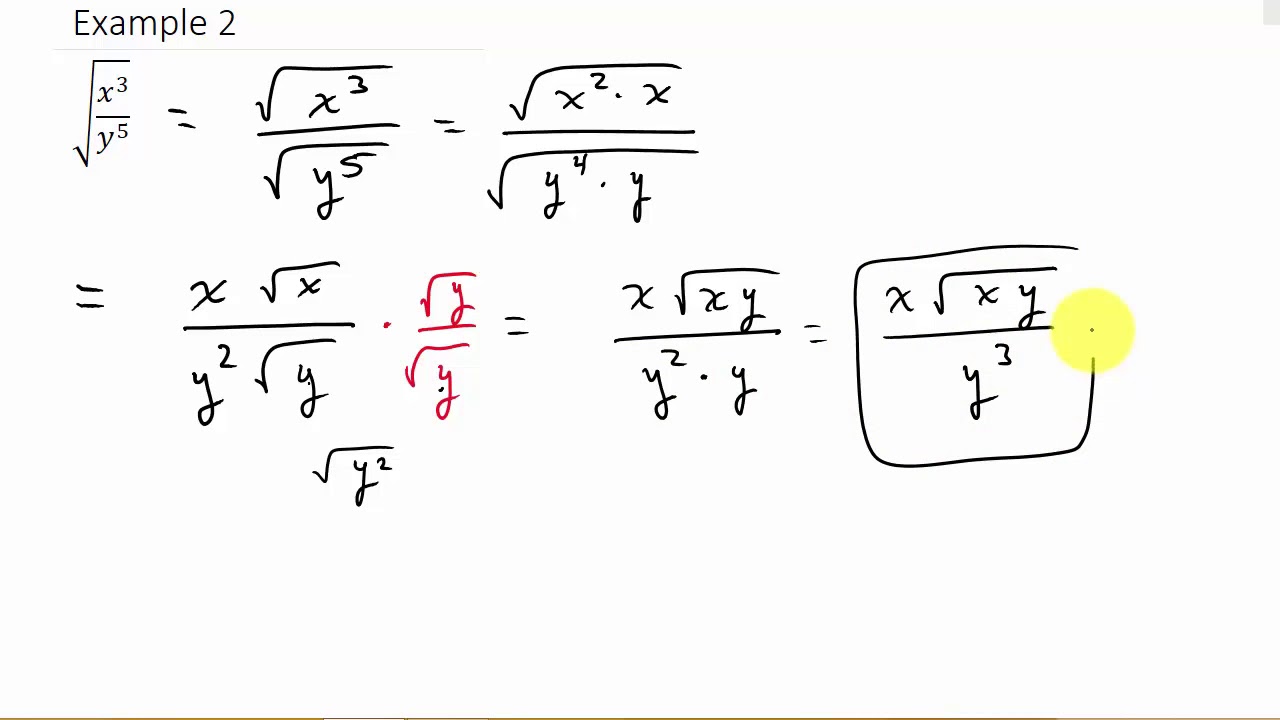
Example 2: Rationalizing a Binomial Denominator

For a fraction like 2/(3 + √2), you can rationalize it by multiplying by the conjugate of the denominator:

1. Multiply by the conjugate: (2(3 - √2))/((3 + √2)(3 - √2)).
2. Simplify both the numerator and denominator: (6 - 2√2)/(9 - 2) = (6 - 2√2)/7.

3. Examples of Rationalizing the Denominator

Let’s look at more examples to reinforce the concept:

• 1/(4 + √3): Multiply by (4 - √3).
• (5√7)/(√8): Multiply by (√8)/(√8).

4. Common Mistakes to Avoid

While rationalizing the denominator, be mindful of these common mistakes:

• Failing to multiply both the numerator and denominator by the same factor.
• Not simplifying the fraction after rationalization.
• Using incorrect conjugates when dealing with binomial denominators.

5. Applications of Rationalizing the Denominator

Rationalizing the denominator is not only important for theoretical purposes but also has practical applications in various fields:

• Engineering: Simplifies calculations for design and analysis.
• Physics: Necessary for solving equations involving roots.
• Finance: Helps in simplifying ratios and rates.

6. Practice Problems

Here are some practice problems to help you master the concept:

• Rationalize the denominator of 5/(2 + √3).
• Rationalize 4/√7.
• Rationalize (√5)/(3 - √2).

7. Tips for Effective Rationalization

To ensure success in rationalizing the denominator, keep these tips in mind:

• Always check your work for simplification.
• Practice with different types of denominators.
• Understand the concept of conjugates for binomial denominators.

8. Conclusion

In summary, rationalizing the denominator is a vital skill in mathematics that enhances your ability to work with fractions. By following the steps outlined in this article and practicing regularly, you can become proficient at this technique. Don’t hesitate to leave a comment below if you have any questions or share this article with others who may benefit from it. Happy learning!

We hope you found this guide helpful and informative. For more articles on mathematics and related topics, feel free to explore our site. We look forward to seeing you again!